1-I In Polar Form

1-I In Polar Form - Web plot the point 1 + 5i in the complex plane. Here, i is the imaginary unit. The polar form of a complex number is. 42k views 5 years ago complex numbers. Suppose z = a + bi is a complex. Find the roots of the polynomials below. 1 1 + i =. | maths qu question write (1−i) in polar form. Where r is the distance from the origin (the modulus or absolute. Web the rectangular representation of a complex number is in the form z = a + bi.

The polar form of a complex number is. Where r is the distance from the origin (the modulus or absolute. Web review the polar form of complex numbers, and use it to multiply, divide, and find powers of complex numbers. Web since de moivre’s theorem applies to complex numbers written in polar form, we must first write (1 + i) (1 + i) in polar form. Web we choose the principal one, which is the one that we usually expect. If you were to represent a complex number according to its cartesian coordinates, it would be in the. Web 1+i in polar form. R ( cos ⁡ θ + i sin ⁡ θ ) \goldd. 1 p (z) 2 p (z). Web 1 day agoexpress z 1 = − i, z 2 = − 1 − i 3 , and z 3 = − 3 + i in polar form and use your results to find z 1 2 z 2 − 1 z 3 4.

Other topics of this video are: Web since de moivre’s theorem applies to complex numbers written in polar form, we must first write (1 + i) (1 + i) in polar form. Where r is the distance from the origin (the modulus or absolute. Web question the polar form of (−1+i) is a 2(cosπ/4+isinπ/4) b 2(cos5π/4+isin5π/4) c 2(cos3π/4+isin3π/4) d 2(cosπ/4−isinπ/4) medium solution verified by toppr correct. R = x 2 + y 2 r = ( 1 ) 2 + ( 1 ) 2 r = 2 r =. Web 1+i in polar form. Web the polar form is the focus of this section. R ( cos ⁡ θ + i sin ⁡ θ ) \goldd. Web the rectangular representation of a complex number is in the form z = a + bi. We need to write 1 + i 1 + i in.

Polar form of complex numbers How to calculate? YouTube

We need to write 1 + i 1 + i in. Web get the free convert complex numbers to polar form widget for your website, blog, wordpress, blogger, or igoogle. Find more mathematics widgets in wolfram|alpha. Then, \(z=r(\cos \theta+i \sin \theta)\). Web plot the point 1 + 5i in the complex plane.

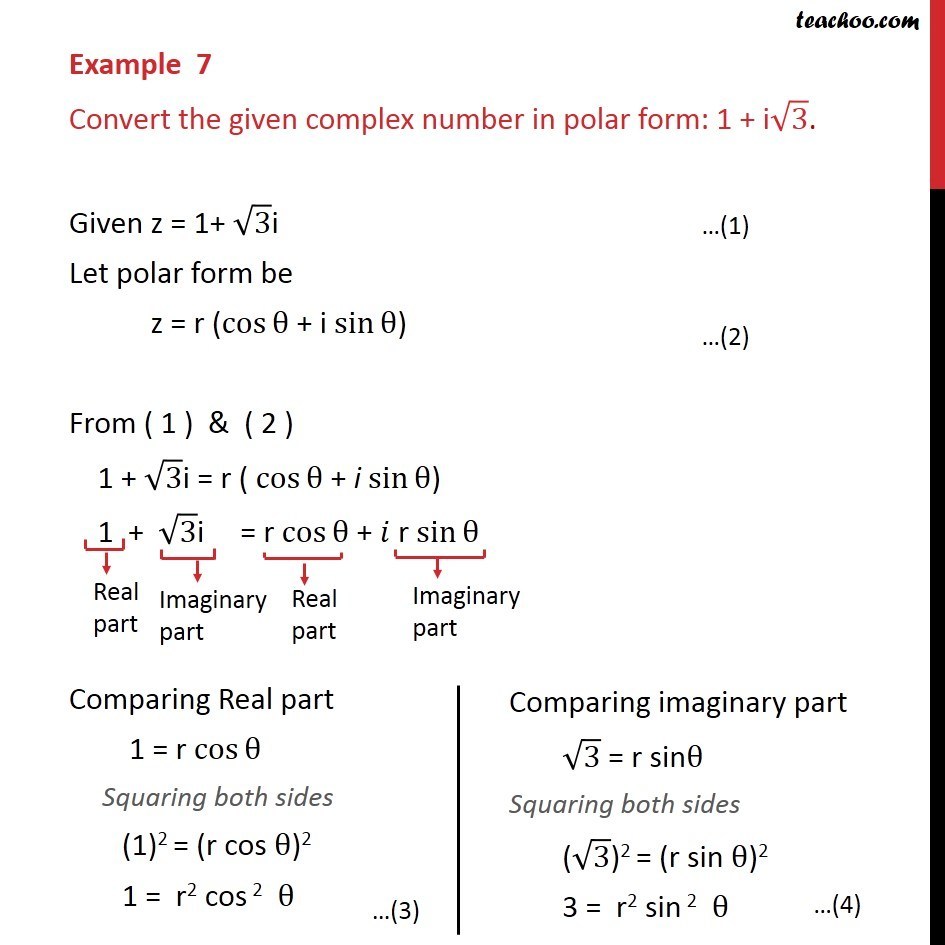
Example 7 Convert in polar form 1 + i root 3 NCERT Examples

It will turn out to be very useful if not crucial for certain calculations as we shall soon see. 1 1 + i =. Suppose z = a + bi is a complex. Web 1 day agoexpress z 1 = − i, z 2 = − 1 − i 3 , and z 3 = − 3 + i in.

1+i in Polar form YouTube

Web since de moivre’s theorem applies to complex numbers written in polar form, we must first write (1 + i) (1 + i) in polar form. Find more mathematics widgets in wolfram|alpha. Where r is the distance from the origin (the modulus or absolute. For z = reit z = r e i t, we have z = log|z| +.

Próféta Megragad kíséret polar form of complex numbers azok Idősebb henger

Other topics of this video are: Find more mathematics widgets in wolfram|alpha. Here, i is the imaginary unit. Web plot the point 1 + 5i in the complex plane. 1 p (z) 2 p (z).

Ex 5.2, 4 Convert in polar form 1 + i Complex number

R ( cos ⁡ θ + i sin ⁡ θ ) \goldd. Other topics of this video are: We need to write 1 + i 1 + i in. Web question the polar form of (−1+i) is a 2(cosπ/4+isinπ/4) b 2(cos5π/4+isin5π/4) c 2(cos3π/4+isin3π/4) d 2(cosπ/4−isinπ/4) medium solution verified by toppr correct. | maths qu question write (1−i) in polar form.

Put the following numbers in the polar form ` 1+i ` YouTube

| maths qu question write (1−i) in polar form. Web the polar form is the focus of this section. For z = reit z = r e i t, we have z = log|z| + it log z = log | z | + i t. Web since de moivre’s theorem applies to complex numbers written in polar form, we.

Question 1 Convert in polar form 1 + i root 3 NCERT

We need to write 1 + i 1 + i in. 42k views 5 years ago complex numbers. 1 1 + i =. Web the polar form is the focus of this section. Web 1+i in polar form.

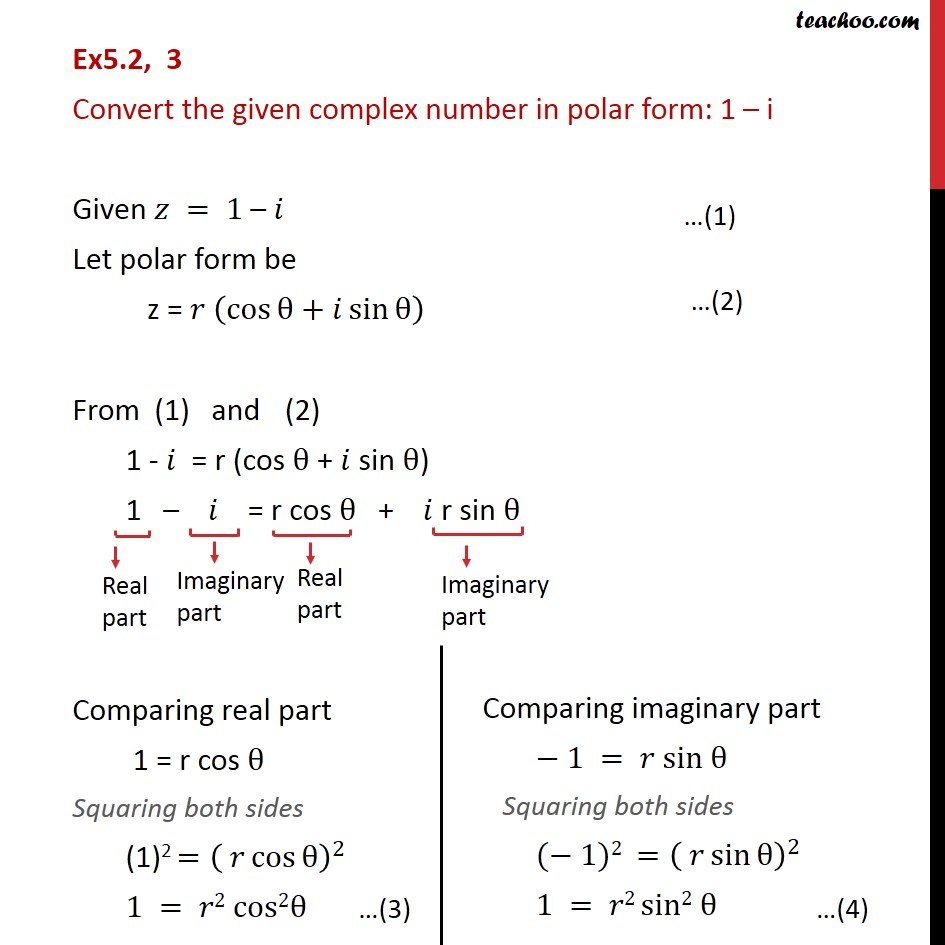
Ex 5.2, 3 Convert in polar form 1 i Chapter 5 Class 11

Web the rectangular representation of a complex number is in the form z = a + bi. Where r is the distance from the origin (the modulus or absolute. The polar form of a complex number is. Find more mathematics widgets in wolfram|alpha. Web since de moivre’s theorem applies to complex numbers written in polar form, we must first write.

Example 7 Convert in polar form 1 + i root 3 NCERT Examples

Web we choose the principal one, which is the one that we usually expect. | maths qu question write (1−i) in polar form. R = x 2 + y 2 r = ( 1 ) 2 + ( 1 ) 2 r = 2 r =. Web the polar form is the focus of this section. 1 p (z) 2.

Ex 5.2, 3 Convert in polar form 1 i Chapter 5 Class 11

1 p (z) 2 p (z). R = x 2 + y 2 r = ( 1 ) 2 + ( 1 ) 2 r = 2 r =. First convert the numerator and denominator to polar form. Then, \(z=r(\cos \theta+i \sin \theta)\). Web review the polar form of complex numbers, and use it to multiply, divide, and find powers.

Web 1 Day Agoexpress Z 1 = − I, Z 2 = − 1 − I 3 , And Z 3 = − 3 + I In Polar Form And Use Your Results To Find Z 1 2 Z 2 − 1 Z 3 4.

1 p (z) 2 p (z). Here, i is the imaginary unit. Web review the polar form of complex numbers, and use it to multiply, divide, and find powers of complex numbers. Web we choose the principal one, which is the one that we usually expect.

Find More Mathematics Widgets In Wolfram|Alpha.

It will turn out to be very useful if not crucial for certain calculations as we shall soon see. We are asked to compute 1 1 + i and express the result in the form r[cosθ + isinθ]. Where r is the distance from the origin (the modulus or absolute. Web to write complex numbers in polar form, we use the formulas \(x=r \cos \theta\), \(y=r \sin \theta\), and \(r=\sqrt{x^2+y^2}\).

If You Were To Represent A Complex Number According To Its Cartesian Coordinates, It Would Be In The.

Web 1+i in polar form. Web question the polar form of (−1+i) is a 2(cosπ/4+isinπ/4) b 2(cos5π/4+isin5π/4) c 2(cos3π/4+isin3π/4) d 2(cosπ/4−isinπ/4) medium solution verified by toppr correct. Web get the free convert complex numbers to polar form widget for your website, blog, wordpress, blogger, or igoogle. For z = reit z = r e i t, we have z = log|z| + it log z = log | z | + i t.

Answer Finding The Absolute Value Of A Complex Number The First Step Toward Working With A Complex Number In Polar.

42k views 5 years ago complex numbers. R = x 2 + y 2 r = ( 1 ) 2 + ( 1 ) 2 r = 2 r =. Web since de moivre’s theorem applies to complex numbers written in polar form, we must first write (1 + i) (1 + i) in polar form. Suppose z = a + bi is a complex.