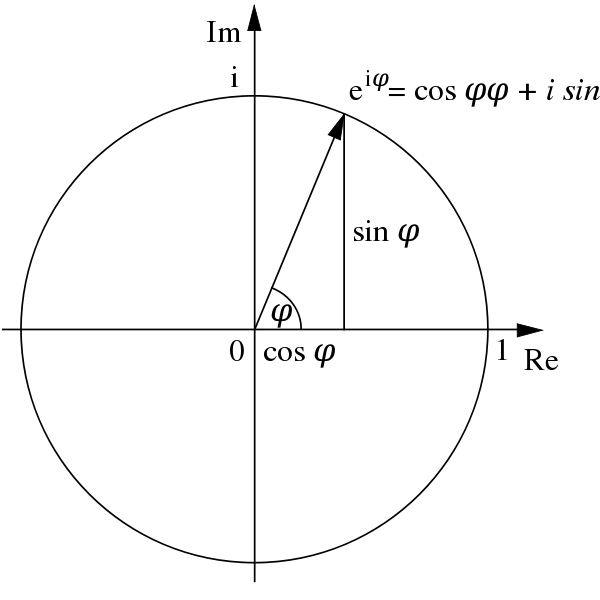
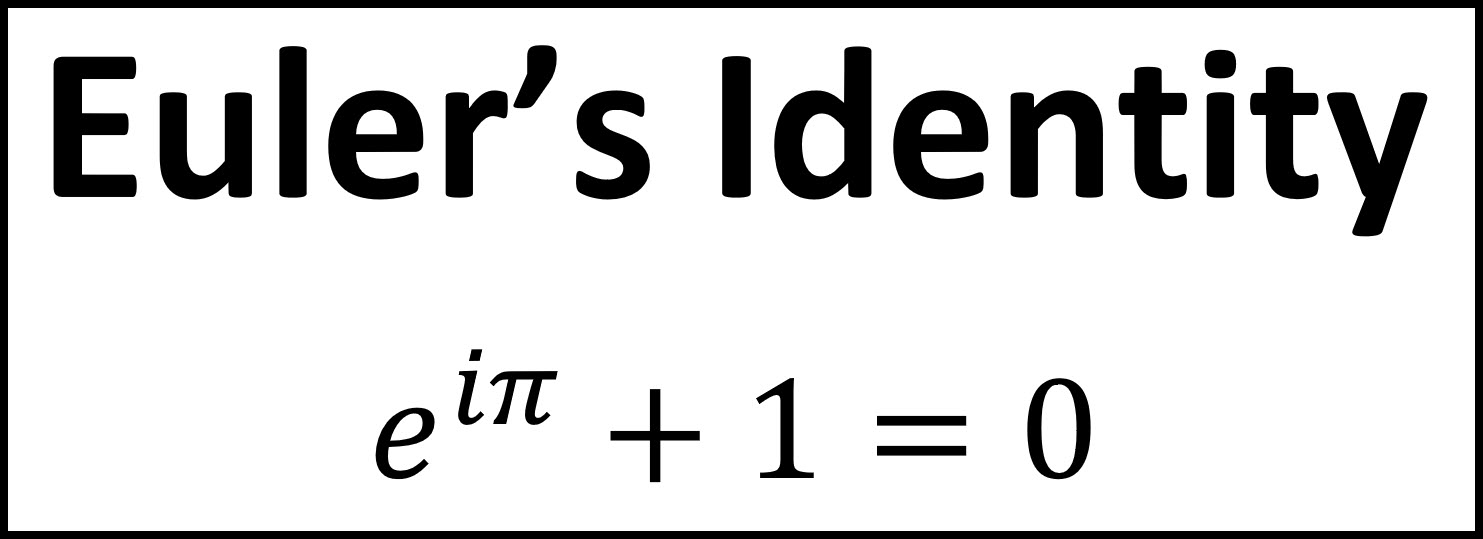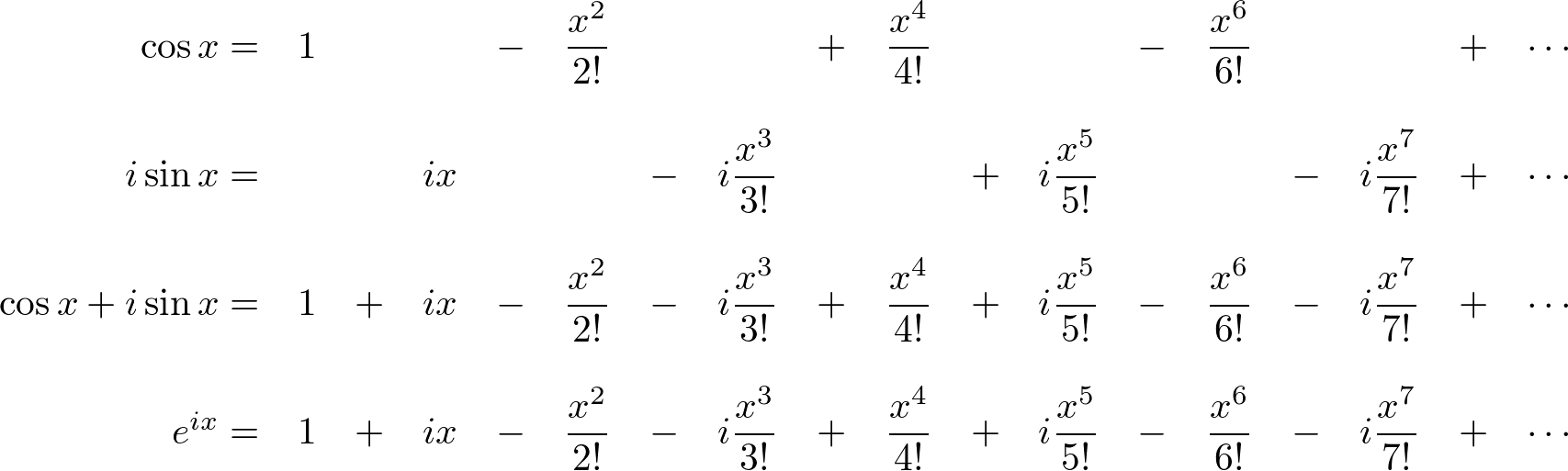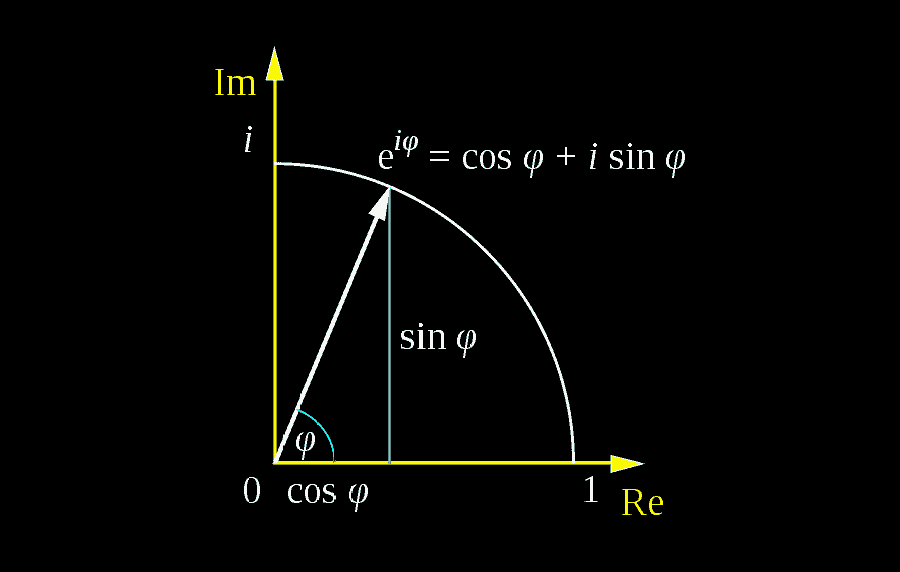Cosine In Euler Form
Cosine In Euler Form - The complex plane complex numbers are represented geometrically by points in the plane: Web answer (1 of 9): Web sine and cosine are written as sums of complex exponentials. Web euler’s formula, polar representation 1. It turns messy trig identities into tidy rules for. Web euler's formula can be used to prove the addition formula for both sines and cosines as well as the double angle formula (for the addition formula, consider $\mathrm{e^{ix}}$. Suppose we have a function ∠\theta=\cos\theta+i\sin\theta; Web euler's formula is a relationship between exponents of imaginary numbers and the trigonometric functions: It is why electrical engineers need to. Web euler's formula relates the complex exponential to the cosine and sine functions.
Web finally, there is a nice formula discovered by leonhard euler in the 1700s that allows us to relate complex numbers, trigonometric functions and exponents into one single formula:. The simple derivation uses euler's formula. It turns messy trig identities into tidy rules for. It is why electrical engineers need to. For example, if , then relationship to sin and cos in euler's. Web sine and cosine emerge from vector sum of three spinning numbers in euler’s formula, the green spinning number is. The identities are useful in simplifying equations. The complex plane complex numbers are represented geometrically by points in the plane: The hyperbolic sine and the hyperbolic cosine. Web in complex analysis, the hyperbolic functions arise when applying the ordinary sine and cosine functions to an imaginary angle.
For example, if , then relationship to sin and cos in euler's. Using these formulas, we can. It turns messy trig identities into tidy rules for. This formula is the most important tool in ac analysis. Web euler's formula can be used to prove the addition formula for both sines and cosines as well as the double angle formula (for the addition formula, consider $\mathrm{e^{ix}}$. Web euler's formula is a relationship between exponents of imaginary numbers and the trigonometric functions: The simple derivation uses euler's formula. It is why electrical engineers need to. Web answer (1 of 9): Web euler’s formula, polar representation 1.
Half Angles from Euler's Formula
Web euler's formula is a relationship between exponents of imaginary numbers and the trigonometric functions: The complex plane complex numbers are represented geometrically by points in the plane: Web sine and cosine are written as sums of complex exponentials. Let me try this from a different angle: Web euler's formula for product of cosines asked 7 years, 7 months ago.
Euler's Formula
Web sine and cosine emerge from vector sum of three spinning numbers in euler’s formula, the green spinning number is. Web in complex analysis, the hyperbolic functions arise when applying the ordinary sine and cosine functions to an imaginary angle. {\displaystyle e^{ix}=\cos x+i\sin x.} this formula is commonly considered for real values. Web euler's formula is a relationship between exponents.
Euler's Equation
Suppose we have a function ∠\theta=\cos\theta+i\sin\theta; It turns messy trig identities into tidy rules for. E i x = cos x + i sin x. Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒.
Relationship between sine, cosine and exponential function
Web in complex analysis, the hyperbolic functions arise when applying the ordinary sine and cosine functions to an imaginary angle. The complex plane complex numbers are represented geometrically by points in the plane: It is why electrical engineers need to. The number a + ib is represented by the. Web euler's formula can be used to prove the addition formula.
Trigonometric Form Into A Complex Number
The complex plane complex numbers are represented geometrically by points in the plane: It is why electrical engineers need to. The simple derivation uses euler's formula. The number a + ib is represented by the. {\displaystyle e^{ix}=\cos x+i\sin x.} this formula is commonly considered for real values.
Direction Cosine Matrix Euler Integration Failure YouTube
It is why electrical engineers need to. For example, if , then relationship to sin and cos in euler's. Web euler's formula relates sine and cosine to the exponential function: Web euler’s (pronounced ‘oilers’) formula connects complex exponentials, polar coordinates, and sines and cosines. Web euler's formula is a relationship between exponents of imaginary numbers and the trigonometric functions:
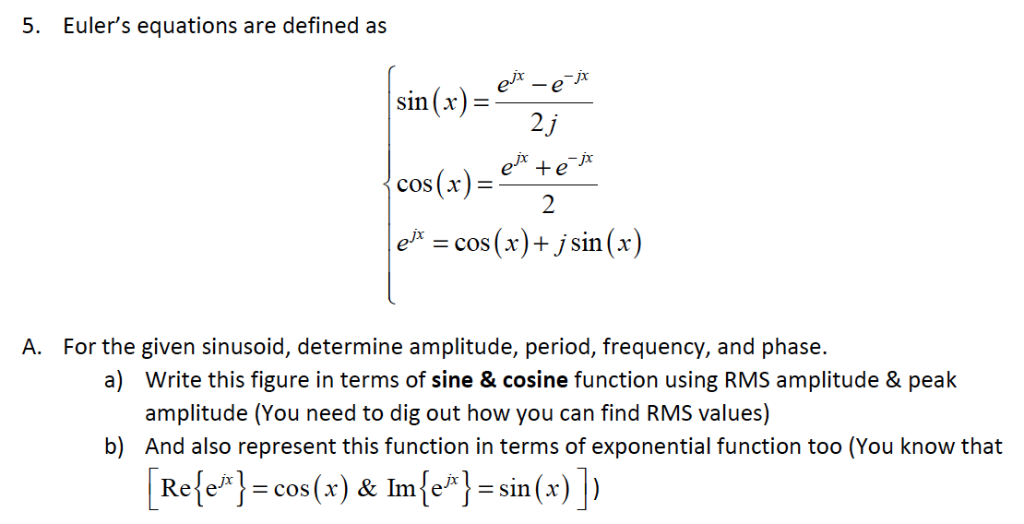
Solved 5. Euler's equations are defined as sin (x) cos(x) e"
Web v t e in mathematics, euler's identity [note 1] (also known as euler's equation) is the equality where e is euler's number, the base of natural logarithms, i is the imaginary unit, which. Web euler's formula can be used to prove the addition formula for both sines and cosines as well as the double angle formula (for the addition.
Euler's cosine wave YouTube
Web in complex analysis, the hyperbolic functions arise when applying the ordinary sine and cosine functions to an imaginary angle. Web finally, there is a nice formula discovered by leonhard euler in the 1700s that allows us to relate complex numbers, trigonometric functions and exponents into one single formula:. Web sine and cosine emerge from vector sum of three spinning.
Deriving the famous Euler’s formula through Taylor Series Muthukrishnan
Using these formulas, we can. The number a + ib is represented by the. It is why electrical engineers need to. The complex plane complex numbers are represented geometrically by points in the plane: It turns messy trig identities into tidy rules for.
FileEuler's formula.svg New World Encyclopedia
Web euler’s formula, polar representation 1. The hyperbolic sine and the hyperbolic cosine. For example, if , then relationship to sin and cos in euler's. The complex plane complex numbers are represented geometrically by points in the plane: Web finally, there is a nice formula discovered by leonhard euler in the 1700s that allows us to relate complex numbers, trigonometric.
Web In Complex Analysis, The Hyperbolic Functions Arise When Applying The Ordinary Sine And Cosine Functions To An Imaginary Angle.
Web euler’s formula, polar representation 1. Web euler's formula for product of cosines asked 7 years, 7 months ago modified 1 year, 10 months ago viewed 2k times 4 according to squaring the circle by ernest. The number a + ib is represented by the. Web v t e in mathematics, euler's identity [note 1] (also known as euler's equation) is the equality where e is euler's number, the base of natural logarithms, i is the imaginary unit, which.
It Is Why Electrical Engineers Need To.
Web euler's formula relates sine and cosine to the exponential function: It turns messy trig identities into tidy rules for. Web sine and cosine emerge from vector sum of three spinning numbers in euler’s formula, the green spinning number is. The complex plane complex numbers are represented geometrically by points in the plane:
Web Euler’s (Pronounced ‘Oilers’) Formula Connects Complex Exponentials, Polar Coordinates, And Sines And Cosines.
The hyperbolic sine and the hyperbolic cosine. The simple derivation uses euler's formula. Web euler's formula relates the complex exponential to the cosine and sine functions. Web answer (1 of 9):
Using These Formulas, We Can.
{\displaystyle e^{ix}=\cos x+i\sin x.} this formula is commonly considered for real values. Web euler's formula can be used to prove the addition formula for both sines and cosines as well as the double angle formula (for the addition formula, consider $\mathrm{e^{ix}}$. This formula is the most important tool in ac analysis. The identities are useful in simplifying equations.