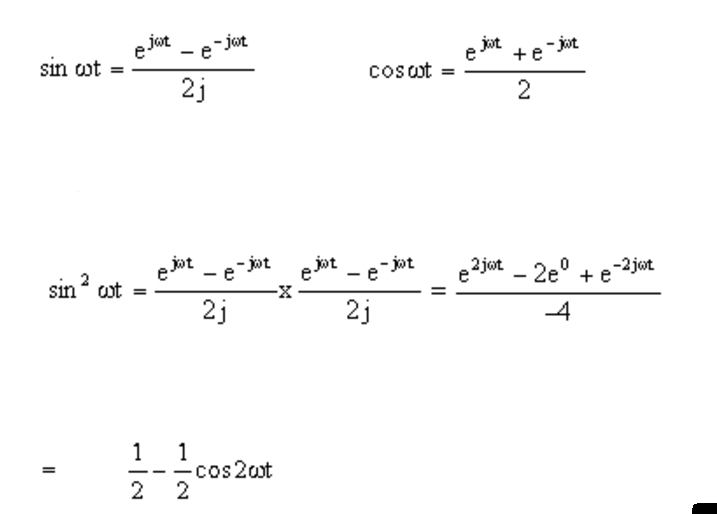
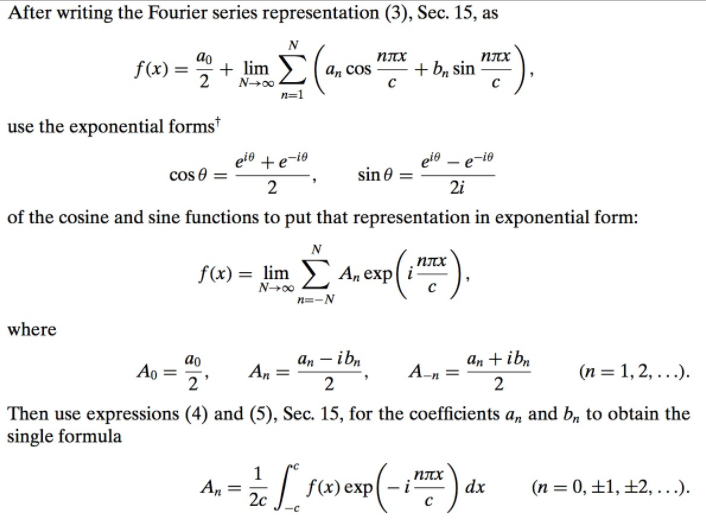
Exponential Form Of Sine And Cosine

Exponential Form Of Sine And Cosine - As a result, the other hyperbolic functions are meromorphic in the whole complex plane. Web the polynomials, exponential function e x, and the trigonometric functions sine and cosine, are examples of entire functions. Web the hyperbolic sine and the hyperbolic cosine are entire functions. Web writing the cosine and sine as the real and imaginary parts of ei , one can easily compute their derivatives from the derivative of the exponential. There are many other uses and examples of this beautiful and. Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒. Using these formulas, we can. Web answer (1 of 3): Originally, sine and cosine were defined in relation to. Web relations between cosine, sine and exponential functions.

Are they related to euler's formula? Examples of functions that are not entire include the. Web which leads to = (cos t + i sin t) (cos (¡t) + i sin (¡t)) = (cos t + i sin t) (cos t ¡ i sin t) = cos2 t ¡ i2 sin2 t = cos2 t + sin2 t: One has d d cos = d d re(ei ) = d. Web relations between cosine, sine and exponential functions. Where do the exponential definitions of sine and cosine from? Using these formulas, we can. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Web specifically, they are the inverses of the sine, cosine, tangent, cotangent, secant, and cosecant functions, and are used to obtain an angle from any of the angle's trigonometric. Web writing the cosine and sine as the real and imaginary parts of ei , one can easily compute their derivatives from the derivative of the exponential.

There are many other uses and examples of this beautiful and. Periodicity of the complex sine. Are they related to euler's formula? Originally, sine and cosine were defined in relation to. Web the polynomials, exponential function e x, and the trigonometric functions sine and cosine, are examples of entire functions. Web addition formula for the complex exponential, we see that ei2z = 1, whereupon, by xi, there’s an integer n such that 2z = 2…n, i.e., z = n…. Web answer (1 of 3): Where do the exponential definitions of sine and cosine from? Web the hyperbolic sine and the hyperbolic cosine are entire functions. Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒.

Basics of QPSK modulation and display of QPSK signals Electrical

Web addition formula for the complex exponential, we see that ei2z = 1, whereupon, by xi, there’s an integer n such that 2z = 2…n, i.e., z = n…. Periodicity of the complex sine. Examples of functions that are not entire include the. Where do the exponential definitions of sine and cosine from? There are many other uses and examples.

Function For Sine Wave Between Two Exponential Cuves Mathematics

Originally, sine and cosine were defined in relation to. Web which leads to = (cos t + i sin t) (cos (¡t) + i sin (¡t)) = (cos t + i sin t) (cos t ¡ i sin t) = cos2 t ¡ i2 sin2 t = cos2 t + sin2 t: Using these formulas, we can. Web we can.

EM to Optics 10 Converting Cos & Sine to Complex Exponentials YouTube

Periodicity of the complex sine. Web the polynomials, exponential function e x, and the trigonometric functions sine and cosine, are examples of entire functions. There are many other uses and examples of this beautiful and. Examples of functions that are not entire include the. One has d d cos = d d re(ei ) = d.

EXPONENTIAL FORM OF COMPLEX NUMBERS YouTube

One has d d cos = d d re(ei ) = d. Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒. Web relations between cosine, sine and exponential.

Write Equations Of Sine Functions Using Properties Calculator

Using these formulas, we can. Originally, sine and cosine were defined in relation to. As a result, the other hyperbolic functions are meromorphic in the whole complex plane. Web specifically, they are the inverses of the sine, cosine, tangent, cotangent, secant, and cosecant functions, and are used to obtain an angle from any of the angle's trigonometric. Web which leads.

Question Video Converting the Product of Complex Numbers in Polar Form

Originally, sine and cosine were defined in relation to. Examples of functions that are not entire include the. Web the hyperbolic sine and the hyperbolic cosine are entire functions. Periodicity of the complex sine. Using these formulas, we can.

Other Math Archive January 29, 2018

Web complex exponential definition of sine and cosine qncubed3 7.4k subscribers subscribe 4.2k views 3 years ago today, we derive the complex. Web addition formula for the complex exponential, we see that ei2z = 1, whereupon, by xi, there’s an integer n such that 2z = 2…n, i.e., z = n…. Web writing the cosine and sine as the real.

Relationship between sine, cosine and exponential function

There are many other uses and examples of this beautiful and. Where do the exponential definitions of sine and cosine from? Are they related to euler's formula? One has d d cos = d d re(ei ) = d. As a result, the other hyperbolic functions are meromorphic in the whole complex plane.

complex numbers Converting i to exponential form Mathematics

Web the polynomials, exponential function e x, and the trigonometric functions sine and cosine, are examples of entire functions. Periodicity of the complex sine. There are many other uses and examples of this beautiful and. Web specifically, they are the inverses of the sine, cosine, tangent, cotangent, secant, and cosecant functions, and are used to obtain an angle from any.

Sine and cosine problems Math Tutoring & Exercises

How to find out the sin value. Are they related to euler's formula? Web complex exponential definition of sine and cosine qncubed3 7.4k subscribers subscribe 4.2k views 3 years ago today, we derive the complex. There are many other uses and examples of this beautiful and. Web specifically, they are the inverses of the sine, cosine, tangent, cotangent, secant, and.

Web Specifically, They Are The Inverses Of The Sine, Cosine, Tangent, Cotangent, Secant, And Cosecant Functions, And Are Used To Obtain An Angle From Any Of The Angle's Trigonometric.

Web addition formula for the complex exponential, we see that ei2z = 1, whereupon, by xi, there’s an integer n such that 2z = 2…n, i.e., z = n…. Web complex exponential definition of sine and cosine qncubed3 7.4k subscribers subscribe 4.2k views 3 years ago today, we derive the complex. Originally, sine and cosine were defined in relation to. Web answer (1 of 3):

Where Do The Exponential Definitions Of Sine And Cosine From?

Examples of functions that are not entire include the. Using these formulas, we can. There are many other uses and examples of this beautiful and. Web relations between cosine, sine and exponential functions.

How To Find Out The Sin Value.

Are they related to euler's formula? Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Periodicity of the complex sine.

As A Result, The Other Hyperbolic Functions Are Meromorphic In The Whole Complex Plane.

Web writing the cosine and sine as the real and imaginary parts of ei , one can easily compute their derivatives from the derivative of the exponential. Web which leads to = (cos t + i sin t) (cos (¡t) + i sin (¡t)) = (cos t + i sin t) (cos t ¡ i sin t) = cos2 t ¡ i2 sin2 t = cos2 t + sin2 t: Web the polynomials, exponential function e x, and the trigonometric functions sine and cosine, are examples of entire functions. Web the hyperbolic sine and the hyperbolic cosine are entire functions.