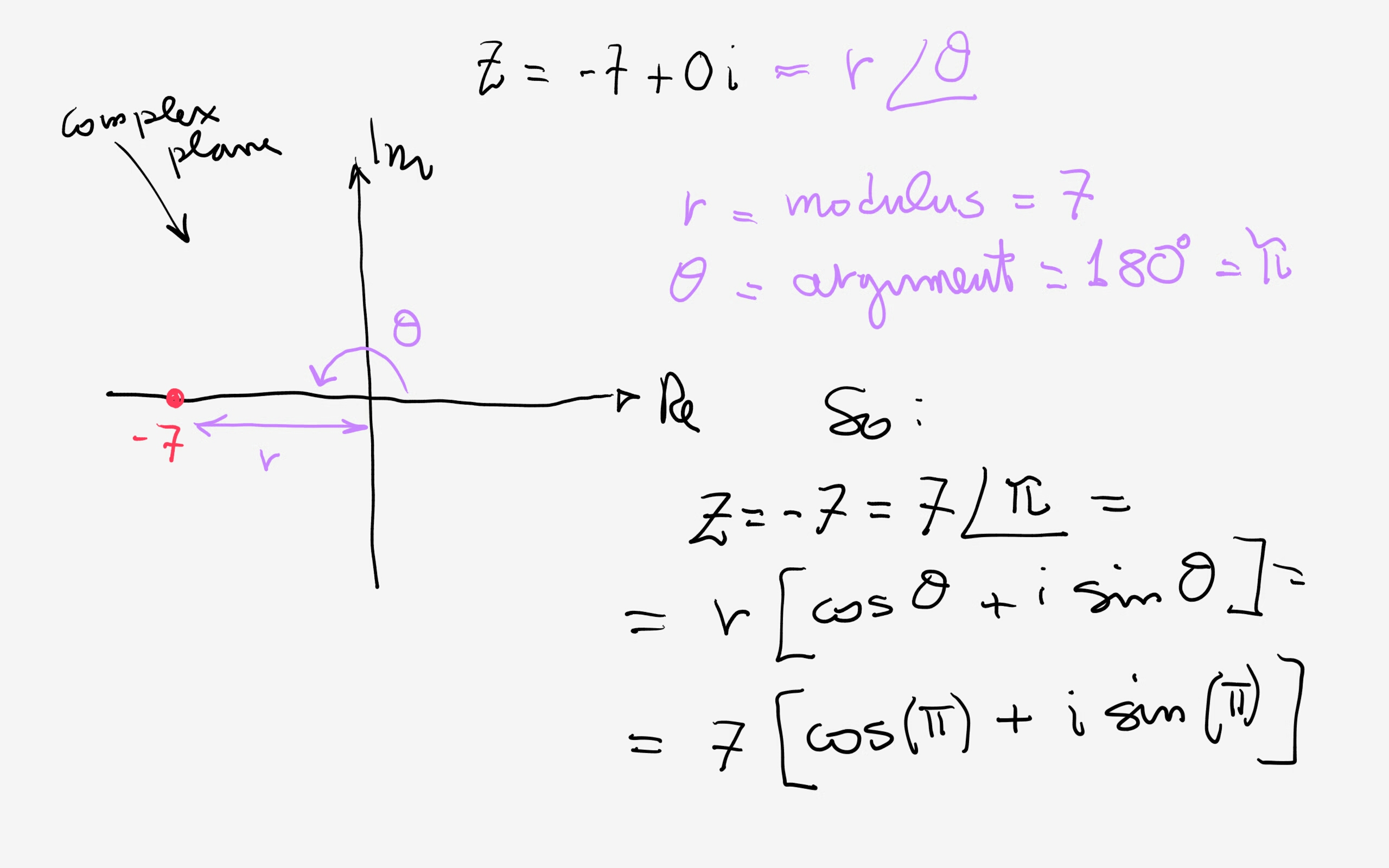Vector In Trigonometric Form
Vector In Trigonometric Form - Web how to write a component form vector in trigonometric form (using the magnitude and direction angle). Want to learn more about vector component form? The vector v = 4 i + 3 j has magnitude. The length of the arrow (relative to some kind of reference or scale) represents the relative magnitude of the vector while the arrow head gives. ˆu = < 2,5 >. Z = a+ bi = |z|(cos(θ)+isin(θ)) z = a + b i = | z | ( cos ( θ) + i sin ( θ)) ‖ v ‖ = 3 2 + 4 2 = 25 = 5. Θ = tan − 1 ( 3 4) = 36.9 ∘. Web what are the three forms of vector? The sum of (1,3) and (2,4) is (1+2,3+4), which is (3,7) show more related symbolab blog posts
This complex exponential function is sometimes denoted cis x (cosine plus i sine). Web where e is the base of the natural logarithm, i is the imaginary unit, and cos and sin are the trigonometric functions cosine and sine respectively. The length of the arrow (relative to some kind of reference or scale) represents the relative magnitude of the vector while the arrow head gives. Web given the coordinates of a vector (x, y), its magnitude is. Write the result in trig form. Web the vector and its components form a right triangle. Web what are the types of vectors? Web to find the direction of a vector from its components, we take the inverse tangent of the ratio of the components: Thus, we can readily convert vectors from geometric form to coordinate form or vice versa. −→ oa = ˆu = (2ˆi +5ˆj) in component form.
Since displacement, velocity, and acceleration are vector quantities, we can analyze the horizontal and vertical components of each using some trigonometry. Both component form and standard unit vectors are used. −→ oa = ˆu = (2ˆi +5ˆj) in component form. Web a vector is defined as a quantity with both magnitude and direction. Web to find the direction of a vector from its components, we take the inverse tangent of the ratio of the components: Web what are the types of vectors? 10 cos120°,sin120° find the component form of the vector representing velocity of an airplane descending at 100 mph at 45° below the horizontal. This is much more clear considering the distance vector that the magnitude of the vector is in fact the length of the vector. Web where e is the base of the natural logarithm, i is the imaginary unit, and cos and sin are the trigonometric functions cosine and sine respectively. The length of the arrow (relative to some kind of reference or scale) represents the relative magnitude of the vector while the arrow head gives.
Vector Components Trigonometry Formula Sheet Math words, Math quotes
How to write a component. Component form in component form, we treat the vector as a point on the coordinate plane, or as a directed line segment on the plane. Then, using techniques we'll learn shortly, the direction of a vector can be calculated. Web how to write a component form vector in trigonometric form (using the magnitude and direction.
Pc 6.3 notes_vectors
Θ = tan − 1 ( 3 4) = 36.9 ∘. Thus, we can readily convert vectors from geometric form to coordinate form or vice versa. Adding vectors in magnitude & direction form. Web where e is the base of the natural logarithm, i is the imaginary unit, and cos and sin are the trigonometric functions cosine and sine respectively..
PPT Introduction to Biomechanics and Vector Resolution PowerPoint
Web this calculator performs all vector operations in two and three dimensional space. ˆu = < 2,5 >. Component form in component form, we treat the vector as a point on the coordinate plane, or as a directed line segment on the plane. Web write the vector in trig form. −→ oa and −→ ob.
Trig Form of a Vector YouTube
Both component form and standard unit vectors are used. The formula is still valid if x is a complex number, and so some authors refer to the more general complex version as euler's. Web where e is the base of the natural logarithm, i is the imaginary unit, and cos and sin are the trigonometric functions cosine and sine respectively..
Trigonometric Form To Polar Form
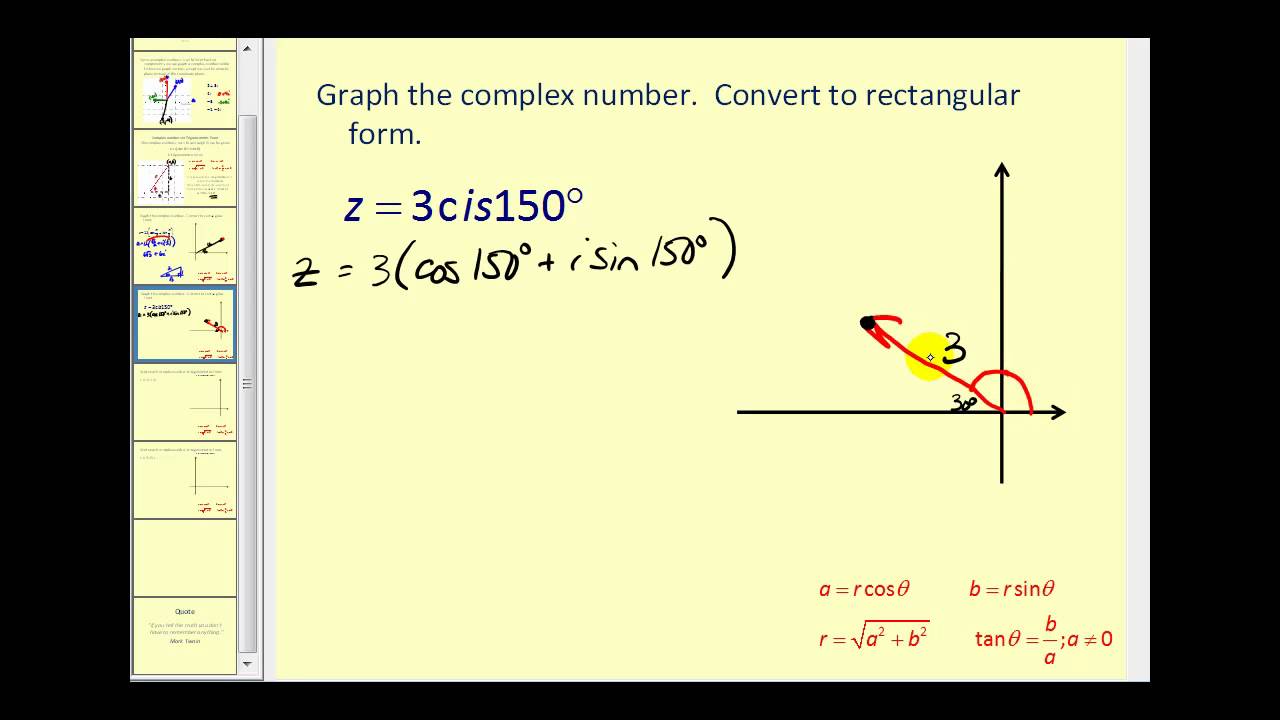
Web a vector is defined as a quantity with both magnitude and direction. This formula is drawn from the **pythagorean theorem* {math/geometry2/specialtriangles}*. Web what are the three forms of vector? Web given the coordinates of a vector (x, y), its magnitude is. Web since \(z\) is in the first quadrant, we know that \(\theta = \dfrac{\pi}{6}\) and the polar form.
Trig Polar/Trigonometric Form of a Complex Number YouTube
10 cos120°,sin120° find the component form of the vector representing velocity of an airplane descending at 100 mph at 45° below the horizontal. −→ oa = ˆu = (2ˆi +5ˆj) in component form. The vector in the component form is v → = 〈 4 , 5 〉. Component form in component form, we treat the vector as a point.
How do you write the complex number in trigonometric form 7? Socratic
Web to find the direction of a vector from its components, we take the inverse tangent of the ratio of the components: Then, using techniques we'll learn shortly, the direction of a vector can be calculated. Z = a+ bi = |z|(cos(θ)+isin(θ)) z = a + b i = | z | ( cos ( θ) + i sin (.
Trigonometric Form To Standard Form
In the above figure, the components can be quickly read. This is much more clear considering the distance vector that the magnitude of the vector is in fact the length of the vector. How do you add two vectors? Web it is a simple matter to find the magnitude and direction of a vector given in coordinate form. Web when.
Vectors in Trigonmetric Form YouTube
ˆu = < 2,5 >. Web when finding the magnitude of the vector, you use either the pythagorean theorem by forming a right triangle with the vector in question or you can use the distance formula. The vector v = 4 i + 3 j has magnitude. Web a vector [math processing error] can be represented as a pointed arrow.
Complex numbers algebraic and trigonometric form GeoGebra
To add two vectors, add the corresponding components from each vector. Web to find the direction of a vector from its components, we take the inverse tangent of the ratio of the components: Web a vector [math processing error] can be represented as a pointed arrow drawn in space: ˆu = < 2,5 >. Then, using techniques we'll learn shortly,.
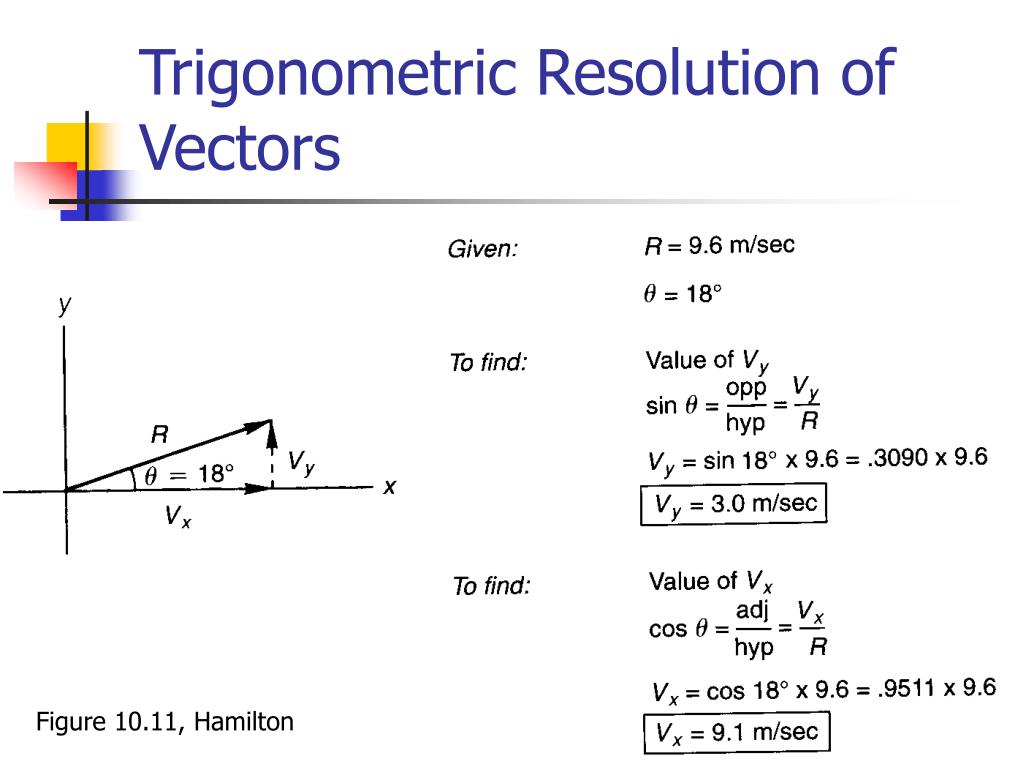
Web The Vector And Its Components Form A Right Angled Triangle As Shown Below.
This is much more clear considering the distance vector that the magnitude of the vector is in fact the length of the vector. Web what are the types of vectors? To add two vectors, add the corresponding components from each vector. In the above figure, the components can be quickly read.
Z = A+ Bi = |Z|(Cos(Θ)+Isin(Θ)) Z = A + B I = | Z | ( Cos ( Θ) + I Sin ( Θ))
Web it is a simple matter to find the magnitude and direction of a vector given in coordinate form. The sum of (1,3) and (2,4) is (1+2,3+4), which is (3,7) show more related symbolab blog posts Web a vector [math processing error] can be represented as a pointed arrow drawn in space: This is the trigonometric form of a complex number where |z| | z | is the modulus and θ θ is the angle created on the complex plane.
Two Vectors Are Shown Below:
The length of the arrow (relative to some kind of reference or scale) represents the relative magnitude of the vector while the arrow head gives. The direction of a vector is only fixed when that vector is viewed in the coordinate plane. How to write a component. You can add, subtract, find length, find vector projections, find dot and cross product of two vectors.
ˆU = < 2,5 >.
This complex exponential function is sometimes denoted cis x (cosine plus i sine). $$v_x = \lvert \overset{\rightharpoonup}{v} \rvert \cos θ$$ $$v_y = \lvert \overset{\rightharpoonup}{v} \rvert \sin θ$$ $$\lvert \overset{\rightharpoonup}{v} \rvert = \sqrt{v_x^2 + v_y^2}$$ $$\tan θ = \frac{v_y}{v_x}$$ Using trigonometry the following relationships are revealed. −12, 5 write the vector in component form.